Geometry, as Usual (II)

H=5

Kobul Chess*

1.d4-d3 g4-g5 2.d3-d2 g5-g6 3.d2-d1=S g6-g7 4.Sd1-b2 g7-g8=B 5.Sb2-c4 Bg8*c4[c1=rS] =

*A King who transforms when a member of his army is captured. When one of his pawn is captured, he changes into traditional King. The Kobul King is always royal. [...]

Geometry, as Usual

Ser=7

Kobul Chess*

1.h7-h5 2.h5-h4 3.h4-h3 4.h3-h2 5.h2-h1=B 6.Bh1-d5 7.Bd5-c4 Sd2*c4[c1=rB] =

*A King who transforms when a memeber of his army is captured. When one of his pawn is captured, he changes into traditional King. When one of his piece (not a Pawn) is captured, he transforms into a piece ofn the same type of the one captured. The Kobul King is always royal. [...]

A Midsummer Night's Pun

Bichrome chess*

h==6

No Kings

1.h7-h6 a2-a3 2.h6-h5 a3-a4 3.h5-h4 a4-a5 4.h4-h3 a5-a6 5.h3-h2 a6-a7 6.h2-h1=B a7-a8=B ==

* All moves, captures and checks must be made between square of opposite colours.A Misdummer n

Twins (or not)

Ser-h=6

Twin Kings

Sentinelles en Pion dverse

Sol. 1.  Kh3 (wPb2) 2. Kh4 (wPb3) 3. Kh5 (wPb4) 4. Kh6 (wPb5) 5. Kh7 (wPb6) 6. Kh8 (wPb7), f8=N =

This problem could be very simplified:

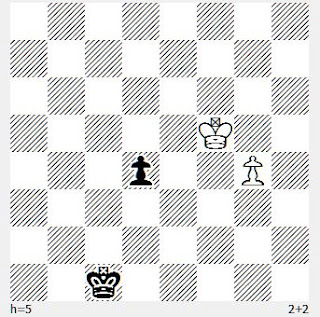
h=5

Sentinelles en pion adverse

No White King

1.Kh3-h4[+wPh3] f2-f4 2.Kh4-h5[+wPh4] f4-f5 3.Kh5-h6[+wPh5] f5-f6 4.Kh6-h7[+wPh6] f6-f7 5.Kh7-h8[+wPh7] f7-f8=S 

Hellzapoppin'

Ser-h#6

pe7 = Royal Pawn*

c1= Fers**

Patrol Chess***

Sentinelles en pion adverse***

No White King

C-

1. e5 2. e4 3. e3 4. e2 5. e1= F 6. Fed2, Sf3#

* The side having this piece is in check if it is threatened.

** A Fers is a fairy chess that moves like a Bishop, but can only go one square.

*** A piece cannot capture or check (but may move or observe) if it is not observed by another piece of its own side.

**** When a piece (not a Pawn) moves, a Pawn of the colour of the opposite side appears on the vacated square, if it is not on the first or the last rank, and if there are less than 8 Pawns of that colour on the board.